Sundays with Sparky - Platonic Solid Eggs...of Science!

Well, I suppose really they're Platonic Solid Eggs of Geometry, or possibly of Engineering, but I have a theme going, you know! :-) This post has its roots in my love of bento, especially Japanese egg molds.  I love them, but can't justify the kitchen space...well, at least not until they make a Tardis egg mold.

Then I saw this post, and realized that of course Japanese housewives didn't start with a unitasker - they have ingenuity at their disposal (I'm betting they don't use octodog cutters, either!)  Looking at their pictures, I realized they were regular polyhedra, also known as the Platonic Solids.  I know of a whole field of geometry (I assume IKEA employs a phalanx of geometricians) for creating flat models, or geometric nets which can be folded into a 3-dimensional shape...and so, the rest, as they say, is history.

I had to do some fancy-footwork math behind-the-scenes before I even broached the subject with Sparky.  I figured out the following: a large egg has a volume of approximately 3.25 tablespoons.  Using Google's excellent calculator function, I found that 3.25 tablespoons equals about 48.05 cubic centimeters (yes, you could use algebra - or you could type "3.25 tablespoons to cubic centimeters" into the google search box.)  Then, using trial-and-error, I approximated the volume using these calculators for a cube, tetrahedron, octahedron and dodecahedron. (I didn't think the icosahedron would mold properly; even the dodecahedron was a bit of a stretch - but we did it because it's our favorite shape.)

I then presented Sparky with these numbers:

• Dodecahedron edge 1.8 cm,  angles 108° =  volume 44.6913 cc (really should be 1.85 for a volume of 48.52 - but who can measure 1/2 a millimeter!  Perfectionists should try a medium egg, instead.)
• Tetrahedron edge 7.4 cm  angles 60° = volume 47.7561cc
• Cube edge: edge 3.6 cm,  angles 90° =  volume 46.656 cc
• Octahedron: edge 4.7 cm,  cc angles 60° = volume 48.9426

And Sparky set to work building the correct size and shape face for each net, using a protractor and a ruler.

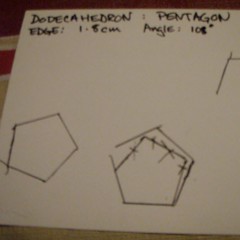
We soon found out how critical it was to have your angles exactly right - we were a fraction of a degree off with the tiny pentagon, and it took us a few tries to get it.  You may think I'm nuts measuring in millimeters, but as the dodecahedron* will show, a small adjustment in edge length means a big variation in volume.

Using the face shapes he cut out, Sparky then made a net model of each solid out of cardstock, which we used as a pattern on our cut-open milk carton (itself, of course, made from a geometric net.)

The next day, Sparky and his friend Meep (welcome to Sundays with Sparky, Meep!) carefully cut out each shape, scored the folds with a ballpoint, folded and assembled it with the tabs on the outside, and I tightly sealed each mold with duct tape, leaving a side open to admit an egg.  To illustrate the symmetry of regular polyhedra, Sparky and Meep decided to wear the same outfit today!

We then made medium-boiled eggs thusly: we put eggs in a saucepan and covered them completely in cold water.  This we brought to a rolling boil.  Immediately, we gave the eggs a vigorous stir to keep the yolk centered, covered the pan, removed it from the heat and allowed it to sit for 9 minutes.  The eggs were then scooped out of the water (do NOT drain the pot,) rinsed and their shells removed (older eggs are easier to peel.) The shelled eggs were dumped back into the hot water to reheat slightly - this keeps them malleable. (This is the reason for medium-boiled eggs; they will continue to cook a bit.)  

While the other eggs waited in the hot water, we quickly popped a hot egg into each mold, slowly closed the cover and squashed it into shape, and then wrapped it vigorously and thoroughly with rubber bands.  The molded eggs went into the refrigerator until completely chilled.

Here is our result! Wrapped:

And Revealed:

We then cut the shaped eggs into quarters (slightly more challenging than one would think!) and made deviled eggs (See - this is why you don't need a deviled egg dish. Just square up your eggs instead!)

One small shallot, minced
Heavy squirt of dijon mustard (about 2 tsp)
About a tablespoon of Greek-style yogurt
About a tablespoon of sour cream
Egg yolks from 3 eggs
Snipped fresh herbs
Salt and pepper to taste

The ingredients were combined in a bowl and blended until creamy.  We then put the mixture into a zip-top bag, snipped a corner, and piped the filling back into the hollow of each egg.

Yum!

In perusing the internet for information on geometric nets, I happened upon Mathematical Food, which has several math-based foodstuffs that seem like tasty fun; I thought I'd give them a shout-out so all my nerd readers can find them!

* At first, I printed a dodecahedron net from the web; it was just 2mm short of the edge length I'd computed.  Note that the egg cracked around the opening and spilled out like an overflowing waffle.  Here are the two dodecahedron molds, the one on the right is the size as computed; almost the correct size: